Class 8 Mathematics
Chapter 6 – Square and Square Roots
Chapter Notes
Properties of the square numbers
(i) A number having 2, 3, 7 or 8 at unit's place is never a perfect square. e.g. 162, 3293 are not perfect squares.
(ii) The number of zeroes at the end of a perfect square is always even. e.g. 100, 400, 10000 are perfect squares.
(iii) The numbers ending in an even number of zeroes may or may not be a perfect square. e.g. 2500 is a perfect square, but 2600 not a perfect square.
(iv) A number ending in an odd number of zeroes is never a perfect square. e.g. 40, 4000 etc., are not perfect squares.
(v) The unit's digit of the square of a natural number is the unit's digit of the square of the digit at the unit's place of given natural number.
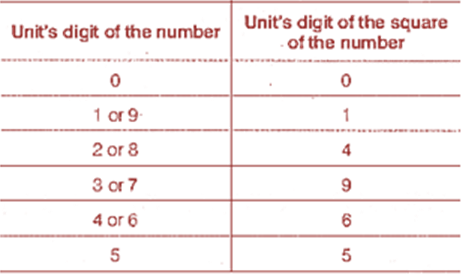
(vi) The squares of odd numbers are odd and the squares of even numbers are even.
e.g. (a) 32 = 9, which are both odd numbers.
(b) 42 = 16, which are both even numbers.
(vii) For every natural number n, the difference of squares of two consecutive natural numbers is equal to their sum i.e. (n + 1)2 – n2 = (n + 1) + n.
e.g. 92 – 82 = 9 + 8 = 17
(viii) There are 2n non–perfect square numbers between the squares of the numbers n and n + 1.
e.g. Natural numbers between 82 and 92 is 2n, i.e. 2 × 8 = 16.
(ix) The square of a natural number n is equal to the sum of first n odd natural numbers.
i.e. n2 = 1 + 3 + 5 + 7 + . . .+ (2n – 1)
So, we can say that the sum of first n odd natural numbers is n2.
e.g. 42 = 1 + 3 + 5 + 7 =· 42 = 16.
(x) A perfect square other than 1 leaves a remainder 0 or 1 when divided by 3 and 4.
e.g. (a) 36 ÷ 3 = 12, remainder = 0
(b) 25 ÷ 4, remainder = 1
(xi) The product of two consecutive even or odd natural numbers is (n2 –1), where n is any natural number i.e. (n + 1) (n – 1) = n2 – 1.
e.g. (a) 11× 13 = 143 = 122 – 1
(b) 12 × 14 = 168 = 132 – 1
Some Patterns
Adding Triangular Numbers
If we combine two consecutive triangular numbers, then we get a dot pattern, which represents a square number i.e.
1 + 3 = 4 = 22 3 + 6 = 9 = 32 6 + 10 = 16 = 42
The square of any odd number as the sum of two consecutive positive integers.
e.g. 32 = 9 = 4 + 5,
where ![]()
Adding 1 to product of consecutive Odd Numbers
If 1 is added to the product of two consecutive odd natural numbers, then it is equal to the square of the only even natural number between them.
i.e. (2n – 1) × (2n + 1) + 1 = 4n2 = (2n)2
Square of Odd Natural Numbers
The square of an odd natural
number, n other than 1 can be expressed as the sum of two consecutive natural numbers ![]() .
.
![]()
Some More Patterns in Square numbers

The squares of the natural numbers like 11, 111, etc. have a pattern
121 × (1 + 2 + 1) = 484 = 222
i.e. 112 × (Sum of digits in 112) = (2 × 11)2
1234321 × (1 + 2 + 3 + 4 + 3 + 2 + 1) = (16 × 1234321) = 19749136 = (4444)2 = (4 × 1111)2
Number like (666667)2, (6666667)2 show following pattern.

Basic rules to find the square of a number:
· Rule I: The square of a number of the form a5 (where, a is ten's digit and 5 is unit's digit) is the number, which ends in 25 and has the number a (a + 1) before 25.
e.g. Let the number be 85.
Here, a = 8 Þ a(a + 1) = 8 × 9 = 72
Hence, 852 = 7225
· Rule II: The square of a number of the form 5a (where, a is unit's digit and 5 is ten's digit) is equal to
(25 + a) × 100 + a2 e.g. Let the number be 56.
Here, a = 6, hence (56)2 = (25 + 6) × 100 + 62 = 3100 + 36 = 3136
· Rule III: The square of three-digit number 5ab (where, b is unit's digit and a is ten's digit) is given by
(5ab)2 = (250 + ab) × 1000 + (ab)2
e.g. Let the number be 527.
Here, a = 2 and, b = 7. Hence, (527)2 = (250 + 27) × 1000 + (27)2 = 277000 + 729 = 277729
· Rule IV: The square of a number abc ... 5 (a number having 5 at unit's place) is obtained by affixing 25 to the right of the number n(n + 1), where n = abc ...
e.g. let the number be 125.
Here, n = 12, then n(n + 1) = 12 × 13 = 156 Hence, 1252 = 15625
Some Other Patterns in Squares
Consider the pattern given below:
(25)2 = 625 = (2 × 3) hundreds + 25
(35)2 = 1225 = (3 × 4) hundreds + 25
(75)2 = 5625 = (7 × 8) hundreds + 25
Thus, for a number with unit's digit 5, i.e. a5, we get (a5)2 = (10a + 5)2 = a(a + 1) hundred + 25
Pythagorean Triplets
A triplet (m, n, p) of three natural numbers m, n and p is called a Pythagorean triplet, if m2 + n2 = p2.
e.g. (3, 4, 5) is Pythagorean triplet, because 32 + 42 = 25 = 52.
For any natural number, m > 1, we have (2m)2 + (m2 – 1)2 = (m2 + 1)2
So, 2m, (m2 – 1) and (m2 + 1) form a Pythagorean triplet.
e.g. One number is 6, then for Pythagorean triplet,
2m = 6 Þ m = 3
m2 – 1 = 32 – 1 = 8 and m2 + 1 = 32 + 1= 10.
So, Pythagorean triplet are 6, 8 and 10.
Note: All Pythagorean triplet may not be obtained using this form. e.g. 5, 12, 13.
Online Tuitions & Self-Study Courses for Grade 6 to 12 & JEE / NEET
